0xDEAFBEEF

BETA: running on Rinkeby Testnet

Artistic Models for Fluid Motion

Observation of nature influences art and science. The mathematics that describe dynamical physical systems are surprisingly compact and elegant. They are generative models. Models of fluid motion are particularly interesting , both mathematically and perceptually. A decade ago(circa 2010), I spent several years studying fluid motion for artistic animation. An academic paper (Full Text PDF) was presented at SIGGRAPH 2012. Accompanying explanation videos can be found here, but please see note at bottom of page to cut to the good parts.

Higher level motivation and background information can be found in this thesis: [Full Text PDF] [M.Sc. Thesis Project Page], excerpts below.

The key insight is that fluid motion can be interpreted as a spectrum, the same way that sound waves are a combination of sinusoidal waves of different frequencies. Sinusoidal waves are like "building blocks" (technically known as eignfunctions). All timbres and sounds can be expressed as combinations of sinusoids of different frequencies.

There is an analogous representation of fluid motion. A set of flow fields can be chosen as building blocks. These flow fields have special properties. Each on their own is a stable flow (technically a stable solution to Navier Stokes equestions). Perceptually, they correspond to spatial scales of vorticity, forming a spectrum.

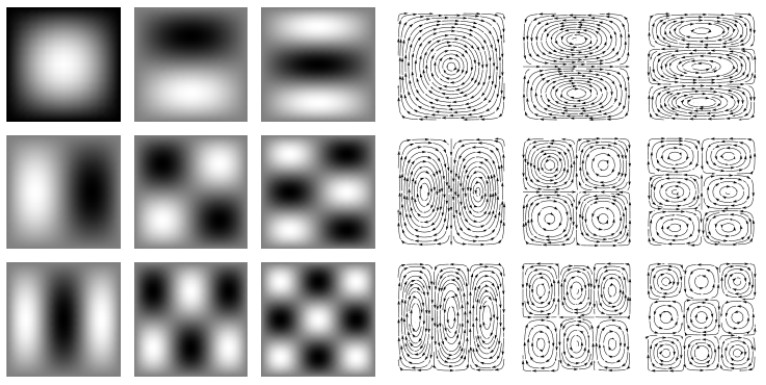
This interpretation provides a number of benefits, including artistic control over some perceptually relevant elements, such as viscosity and energy control in varying parts of the spectrum.

Although not particularly useful in film visual effects, nor of great interest in hard sciences, I was particularly proud of this simple performance based artistic fluid simulation made in Nov 2010. It demonstrates the unique properties of this fluid model.

"Some basis fields exhibit symmetry in one or more spatial directions. The dynamics amongst these basis fields will produce fluid motion that remains symmetric. By initializing an inviscid flow with a few of these basis functions, the resulting dynamics will change perpetually, but always retain an element of symmetry. We use this property along with a few liberal adjustments to design a performance based artistic fluid simulation."

* The complete video submission for the paper are here, and may be instructive, but in hindsight much of it seems tedious. I've selectively edited and presented my favorite parts below (complete with original sloppy harmonica improvisation). This segment was considered outside the scope of research by reviewers, despite it being the most meaningful part personally.